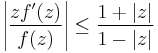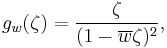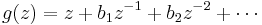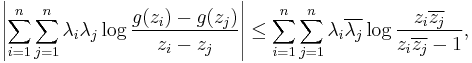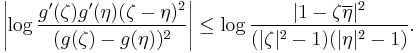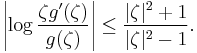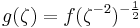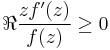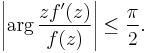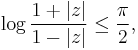Grunsky's theorem

In mathematics, Grunsky's theorem, due to the German mathematician Helmut Grunsky, is a result in complex analysis concerning holomorphic univalent functions defined on the unit disk in the complex numbers. The theorem states that a univalent function defined on the unit disc, fixing the point 0, maps any disk |z| < r onto a starlike domain for r ≤ tanh π/4. The largest r for which this is true is called the radius of starlikeness of the function.

Contents

Statement of theorem

Let f be a univalent holomorphic function on the unit disc D such that f(0) = 0. Then the image of the disc |z| < r is starlike if r ≤ tanh π/4, i.e. it is invariant under multiplication by real numbers in (0,1).

An inequality of Grunsky

If f(z) is univalent on D with f(0) = 0, then

\left|\log {zf^\prime(z)\over f(z)}\right|\le \log {1%2B|z|\over 1-|z|}.

Taking the real and imaginary parts of the logarithm, this implies the two inequalities

\left|{zf^\prime(z)\over f(z)}\right|\le {1%2B|z|\over 1-|z|}

and

\left|\arg {zf^\prime(z)\over f(z)}\right| \le \log {1%2B|z|\over 1-|z|}.

For fixed z, both these equalities are attained by suitable Koebe functions

g_w(\zeta)={\zeta\over (1-\overline{w}\zeta)^2},

where |w| = 1.

Proof of inequality

Grunsky (1932) originally proved these inequalities based on extremal techniques of Ludwig Bieberbach. Subsequent proofs, outlined in Goluzin (1939), relied on the Loewner equation. More elementary proofs were subsequently given based on Goluzin's inequalities, an equivalent form of Grunsky's inequalities (1939) for the Grunsky matrix.

For a univalent function g in z > 1 with an expansion

g(z) = z %2B b_1 z^{-1} %2B b_2 z^{-2} %2B \cdots

Goluzin's inequalities state that

\left|\sum_{i=1}^n\sum_{j=1}^n\lambda_i\lambda_j \log {g(z_i)-g(z_j)\over z_i-z_j}\right| \le \sum_{i=1}^n\sum_{j=1}^n \lambda_i\overline{\lambda_j}\log {z_i\overline{z_j}\over z_i\overline{z_j}-1},

where the zi are distinct points with |zi| > 1 and λi are arbitrary complex numbers.

Taking n = 2. with λ1 = – λ2 = λ, the inequality implies

\left| \log {g^\prime(\zeta)g^\prime(\eta) (\zeta-\eta)^2\over (g(\zeta)-g(\eta))^2}\right|\le \log {|1-\zeta\overline{\eta}|^2\over (|\zeta|^2 -1 )(|\eta|^2 -1)}.

If g is an odd function and η = – ζ, this yields

\left| \log {\zeta g^\prime(\zeta) \over g(\zeta)}\right| \le {|\zeta|^2 %2B 1\over |\zeta|^2 -1}.

Finally if f is any normalized univalent function in D, the required inequality for f follows by taking

g(\zeta)=f(\zeta^{-2})^{-{1\over 2}}

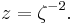
with z=\zeta^{-2}.

Proof of theorem

Let f be a univalent function on D with f(0) = 0. By Nevanlinna's criterion, f is starlike on |z| < r if and only if

\Re {zf^\prime(z)\over f(z)} \ge 0

for |z| < r. Equivalently

\left|\arg {zf^\prime(z)\over f(z)}\right| \le {\pi\over 2}.

On the other hand by the inequality of Grunsky above,

\left|\arg {zf^\prime(z)\over f(z)}\right|\le \log {1%2B|z|\over 1-|z|}.

Thus if

\log {1%2B|z|\over 1-|z|} \le {\pi\over 2},

the inequality holds at z. This condition is equivalent to

|z|\le \tanh {\pi\over 4}

and hence f is starlike on any disk |z| < r with r ≤ tanh π/4.

References